BISAC NAT010000 Ecology
BISAC NAT045050 Ecosystems & Habitats / Coastal Regions & Shorelines
BISAC NAT025000 Ecosystems & Habitats / Oceans & Seas
BISAC NAT045030 Ecosystems & Habitats / Polar Regions
BISAC SCI081000 Earth Sciences / Hydrology
BISAC SCI092000 Global Warming & Climate Change
BISAC SCI020000 Life Sciences / Ecology
BISAC SCI039000 Life Sciences / Marine Biology
BISAC SOC053000 Regional Studies
BISAC TEC060000 Marine & Naval
This study is focused on the alterations and typical features of the wind wave climate of the Black Sea’s coastal waters since 1979 till nowadays. Wind wave parameters were calculated by means of the 3rd-generation numerical spectral wind wave model SWAN, which is widely used on various spatial scales – both coastal waters and open seas. Data on wind speed and direction from the NCEP CFSR reanalysis were used as forcing. The computations were performed on an unstructured computational grid with cell size depending on the distance from the shoreline. Modeling results were applied to evaluate the main characteristics of the wind wave in various coastal areas of the sea.
wave climate, coastal areas, NCEP CFSR reanalysis, SWAN model, climate change
Wind waves are of a crucial importance both to the coastal waters and to the coast itself. They influence nearly all processes occurring in the coastal zone – e.g. marine currents, mixing of freshwater form rivers with marine waters and others. Moreover, wind waves are one of the most hazardous phenomena on the sea and they must be considered during the construction and exploitation of engineering facilities both on the coasts and offshore, shipping and other kinds of human activities. Wind waves are also one of the possible sources of renewable energy.
During the recent decades much efforts were made to improve our understanding of wind waves and corresponding processes in the Black Sea. Wave climate studies corresponding to the entire Black Sea can be found in, e. g., [1], [2]. Another important study subject are storms and their long-term variability [3; 4] as well as waves related to them [5]. Numerous research projects are devoted to the interaction of wind waves with other natural and anthropogenic processes such as wave-current interactions [6], coastal transformations [7] and the influence of waves on the propagation of an oil spill [8]. A practical applications of wind waves is the transformation of their energy into electricity. Therefore, studies of the wave energy potential of the Black Sea and its coastal waters are more and more increasing (e. g. [9], [10] and [11]).
This study is focused on the evolution of parameters of wind waves in the coastal areas of the Black Sea during the recent 37 years. Wind waves were numerically simulated by means of the SWAN model using the NCEP-CFSR reanalysis and an original unstructured computational grid.
I. Data and Methods
Three components are necessary for any numerical study of wind waves – a computation grid, wind data and a numerical model.
The computation grid used in this study is an original unstructured mesh based on digitized nautical maps of the entire Black Sea and its coastal areas. General concepts related to the generation and application of unstructured grids is given in [12] and [13]. The distance between the grid points in this mesh depends on the water depth. In our case this distance varies from 10—15 km in deep-water offshore regions till 500 m in coastal areas (fig. 1, fig. 2). The grid consists of 42284 nodes and 77036 elements. The advantage of such unstructured grids is the possibility to obtain high-resolution data in coastal area and to perform computations in a relatively low amount of grid points at the same time. Previous studies of wind waves on the Black Sea with the use of unstructured grids can be found in e. g. [14] and [15].
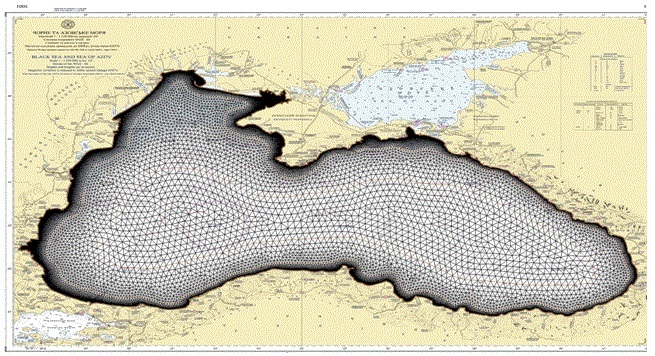
Fig. 1. The entire unstructured computation grid.
High resolution wind fields form the NCEP-CFSR reanalysis [16] were used as forcing for the computations covering the period from 1979 till 2010. For the period form 2011 till 2015 the 2nd version of this reanalysis [17] was used.
The third-generation spectral wind wave model SWAN (version 41.01) [18; 19; 20] was applied for computations of wind wave parameters.
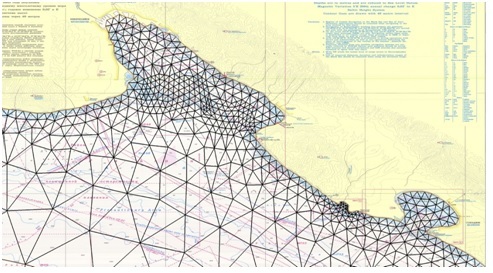
Fig. 2. Part of the unstructured computation grid corresponding to the coastal area between Novorossijsk and Gelendzhik cities (northeastern Black Sea).
The results of computations were validated against data of in situ measurements performed by a Datawell wave buoy. This buoy was installed within the NATO TU-WAVES program 7 km off the city of Gelendzhik (44° 30' 27" N, 37° 58' 42" E). The water depth at the installation point is 85 m. Measurements were carried out from 1996 till 2003 with several interruptions [21; 22].
The comparison of measured and modelled values of significant wave heights (SWH) shows a good agreement between these parameters (fig. 3). The average difference between measured and modelled SWH is 0.20 m; its standard deviation is 0.32 m.

Fig. 3. Scatter plot of measured and modelled SWH at the Gelendzhik buoy.
III. Results and discussion
In this study we analyze timeseries of wind waves parameters corresponding to 5 locations in the coastal waters of the Black Sea – Gelendzhik, Katziveli, the Karkinitskaya Oil Platform, Sinop and Hopa (fig. 4, table 1). Instrumental observations of wind waves within the NATO TU-WAVES project were carried out at all these locations.


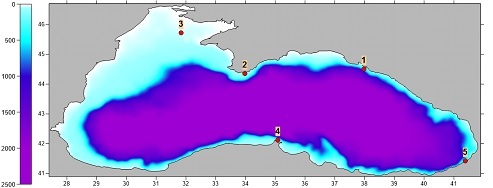
Fig. 4. Bathymetry of the Black Sea and location of study points (1 – Gelendzhik, 2 – Katziveli, 3 - Karkinitskaya Oil Platform, 4 – Sinop, 5 – Hopa).
Table 1. Coordinates of study points
|
Point |
Location |
|
|
1 |
Gelendzhik |
44.5075° N, 37.9783° E |
|
2 |
Katziveli |
44.3557° N, 33.9787° E |
|
3 |
Karkinitskaya Oil Platform |
45.7231° N, 31.8378° E |
|
4 |
Sinop |
42.1233° N, 35.0867° E |
|
5 |
Hopa |
41.4233° N, 41.3833° E |
Some basic statistics of SWH at these location are given in Table 2. Maximal SWH values correspond to Sinop (6.36 m). Maximal SWH at all other study points are at least 0.5 m lower. The lowest SWH maxima correspond to Hopa (4.39 m). The maximal SWH calculated for the Karkinitskaya Oil Platform, which is located at least 50 km off the coast, is also relatively small – 5.35 m. This value is lower than maximal SWH at all other points except Hopa. Such a distribution is in good agreement with the pattern of SWH maxima on the entire Black Sea shown in [1]. According to these results, there are two areas with maximal SWH in the Black Sea – its south-western and north-eastern areas. The relatively shallow north-western area including the Karkinitskaya Oil Platform as well as the south-eastern area including Hopa are the less stormy parts of the sea. Finally, the standard deviation at all locations except Hopa varies between 0.50 and 0.54 m. At the Hopa location it is of 0.40 m.
Table 2. Statistics of SWH in study points
|
Point |
Maximal SWH (m) |
Average SWH (m) |
Standard deviation (m) |
|
Gelendzhik |
5.84 |
0.58 |
0.54 |
|
Katziveli |
5.61 |
0.65 |
0.50 |
|
Karkinitskaya Oil Platform |
5.35 |
0.73 |
0.54 |
|
Sinop |
6.36 |
0.71 |
0.51 |
|
Hopa |
4.39 |
0.47 |
0.40 |
Statistics of wave periods and lengths are given in tab. 3 and tab. 4 correspondingly. Maximal values of these parameters do not vary significantly form point to point. The only exception are these data at the Karkinitskaya Oil platform, where both the maximal wave period (8.03 s) and wave length (83.23 m) are notably lower than at other locations.
Table 3. Statistics of wave periods in study points
|
Point |
Maximal period (s) |
Average period (s) |
Standard deviation (s) |
|
Gelendzhik |
9.44 |
3.28 |
1.47 |
|
Katziveli |
9.71 |
3.60 |
1.25 |
|
Karkinitskaya Oil Platform |
8.03 |
3.26 |
1.08 |
|
Sinop |
9.65 |
3.95 |
1.25 |
|
Hopa |
9.59 |
3.43 |
1.41 |
Table 4. Statistics of wave lengths in study points
|
Point |
Maximal length (m) |
Average length (m) |
Standard deviation (m) |
|
Gelendzhik |
125.57 |
16.02 |
16.24 |
|
Katziveli |
132.20 |
17.24 |
14.15 |
|
Karkinitskaya Oil Platform |
83.23 |
14.82 |
10.85 |
|
Sinop |
128.41 |
21.53 |
15.40 |
|
Hopa |
129.83 |
16.43 |
15.37 |
The distribution of waves depending on their SWH and direction at the study locations is shown on fig. 5. The patterns of these distributions vary significantly form point to point. Thus, two predominant directions of waves can be derived in Gelendzhik – SSE and WNW. Another interesting feature of this distribution is a weak peak corresponding to NW. This peak may be induced by local orographic wind circulation common for the region between the cities of Novorossijsk and Tuapse on the northeastern coast of the sea [23]. In Katziveli there are also two predominant directions of waves – E and WSW. Unlike the pattern in Gelendzhik, there are practically no waves arriving from the shore. Southern waves are very rare as well. As the Karkinitskaya Oil Platform is located far from any coasts the pattern of the directional distribution is more uniform here. However, there are also two prevalent wave directions, namely the SSW and ENE ones. The amount of SE waves is very low here. At the Sinop location there are also two peaks in the distribution of wave directions, namely NW and NNE. Finally, the most evident peak among all these distributions is observed in Hopa, where at least 50% of all waves arrive from WNW.
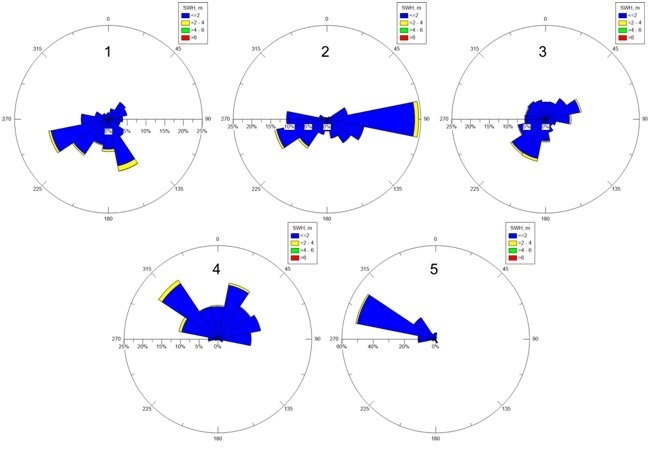
Fig. 5. Wave roses at the study locations. Numbers indicating study points correspond to fig. 4.
IV. Acknowledgments
The reported study was carried out by F.N. Gippius and E.V. Stoliarova within the project № 16-35-00488 funded by the Russian Foundation for Basic Research (RFBR) and by S.A. Myslenkov and V.S. Arkhipkin within the project № 16-08-00829 funded by RFBR.
1. V.S. Arkhipkin, F.N. Gippius, K.P. Koltermann, and G.V. Surkova, “Wind waves in the Black Sea: Results of a hindcast study”, Nat. Hazards Earth Syst. Sci., vol. 14, pp. 2883-2897, November 2014.
2. A. Akpinar, G. Ph. van Vledder, and B. Bingölbali, “Wave climate simulation for the Black Sea basin”, E-proceedings of the 36th IAHR World Congress 28 June - 3 July, 2015, The Hague, the Netherlands.
3. N.N. Valchev, E.V. Trifonova, and N.K. Andreeva, “Past and recent trends in the western Black Sea storminess”, Nat. Hazards Earth Syst. Sci., vol. 12, pp. 961-977, April 2012.
4. G.V. Surkova, V.S. Arkhipkin, and A.V. Kislov, “Atmospheric circulation and storm events in the Black Sea and Caspian Sea”, Central European Journal of Geosciences, vol. 5 (4), pp. 548-559, 2013.
5. V. Galabov, A. Kortcheva, A. Bogatchev, and B. Tsenova, “Investigation of the hydro-meteorological hazards along the bulgarian coast of the Black Sea by reconstructions of historical storms”, Journal of Environmental Protection and Ecology, vol. 16, pp. 1005-1015, 2015.
6. E. Rusu, “Modelling of wave-current interactions at the mouths of the Danube”, J. Mar. Sci. Technol., vol. 15, pp. 143-159, 2010.
7. S. Dan, M.J.F. Stive, D.-J.R.Walstra, and N. Panin, “Wave climate, coastal sediment budget and shoreline changes for the Danube Delta”, Marine Geology, vol. 262, pp. 39-49, 2009.
8. L. Rusu, “Application of numerical models to evaluate oil spills propagation in the coastal environment of the Black Sea”, Journal of Environmental Engineering and Landscape Management, vol. 18 (4), pp. 288-295, December 2010.
9. A. Akpinar, and M.T. Kömürcü, “Wave energy potential along the south-east coasts of the Black Sea”, Energy, vol. 42 (1), pp. 289-302, 2012.
10. H. Keskin Citiroglu, and A. Okur,” An approach to wave energy converter applications in Eregli on the western Black Sea coast of Turkey”, Applied Energy, vol. 135, pp. 738-747, 2014.
11. V.S. Arkhipkin et al., “Assessing the potential of wave energy in coastal waters of Crimea peninsula”, International scientific journal for alternative energy and ecology, vol. 20, pp. 25-35, 2015 (in Russian).
12. J.C. Dietrich et al., “Performance of the unstructured-mesh, SWAN+ADCIRC model in computing hurricane waves and surge”, Journal of Scientific Computing, vol. 52 (2), pp. 468-497, 2012
13. M. Zijlema, “Computation of wind-wave spectra in coastal waters with SWAN on unstructured grids”, Coast. Eng., vol. 57, pp. 267-277, 2010.
14. S.A. Myslenkov, and V.S. Arkhipkin, “Analysis of wind waves in the Bay of Cemes of the Black Sea by means of the SWAN model”, Proceedings of Hydrometcentre of Russia, vol. 350, pp. 58-67, 2013 (in Russian).
15. E.V. Stoliarova, and S.A. Myslenkov, “High resolution wave forecast system in Kerch strait”, Proceedings of Hydrometcentre of Russia, vol. 354, pp. 24-35, 2015 (in Russian).
16. S. Saha et al., “The NCEP climate forecast system reanalysis”, Bulletin of the American Meteorological Society, vol. 91 (8), pp. 1015-1057, 2010.
17. S. Saha et al., “The NCEP climate forecast system version 2”, Journal of Climate, vol. 27 (6), pp. 2185-2208, 2014.
18. N. Booij, R.C. Ris, and L.H. Holthuijsen, ”A third-generation wave model for coastal regions 1. Model description and validation”, Journal of Geophysical Research C: Oceans, vol. 104 (C4), pp. 7649-7666, 1999.
19. R.C. Ris, L.H. Holthuijsen, and N. Booij, “A third-generation wave model for coastal regions 2. Verification”, Journal of Geophysical Research C: Oceans, vol. 104 (C4), pp. 7667-7681, 1999.
20. SWAN Technical Documentation, SWAN Cycle III version 40.51A. Delft: University of Technology, 2007, 98 p.
21. A.V. Boukhanovsky, B.V. Divinsky, R.D. Kos’yan, L.I. Lopatoukhin, and V.A. Rozhkov, “Typification of wind disturbance of the Black Sea under the instrumental data”, Oceanology, vol. 40 (2), pp. 289-297, 2000 (in Russian).
23. P.A. Toropov, S.A. Myslenkov, and T.E. Samsonov, “Numerical modeling of bora in novorossiysk and associated wind waves”, Vestnik Moskovskogo Universiteta, Seriya 5: Geografiya, vol. 2, pp. 38-46, 2013 (in Russian).







